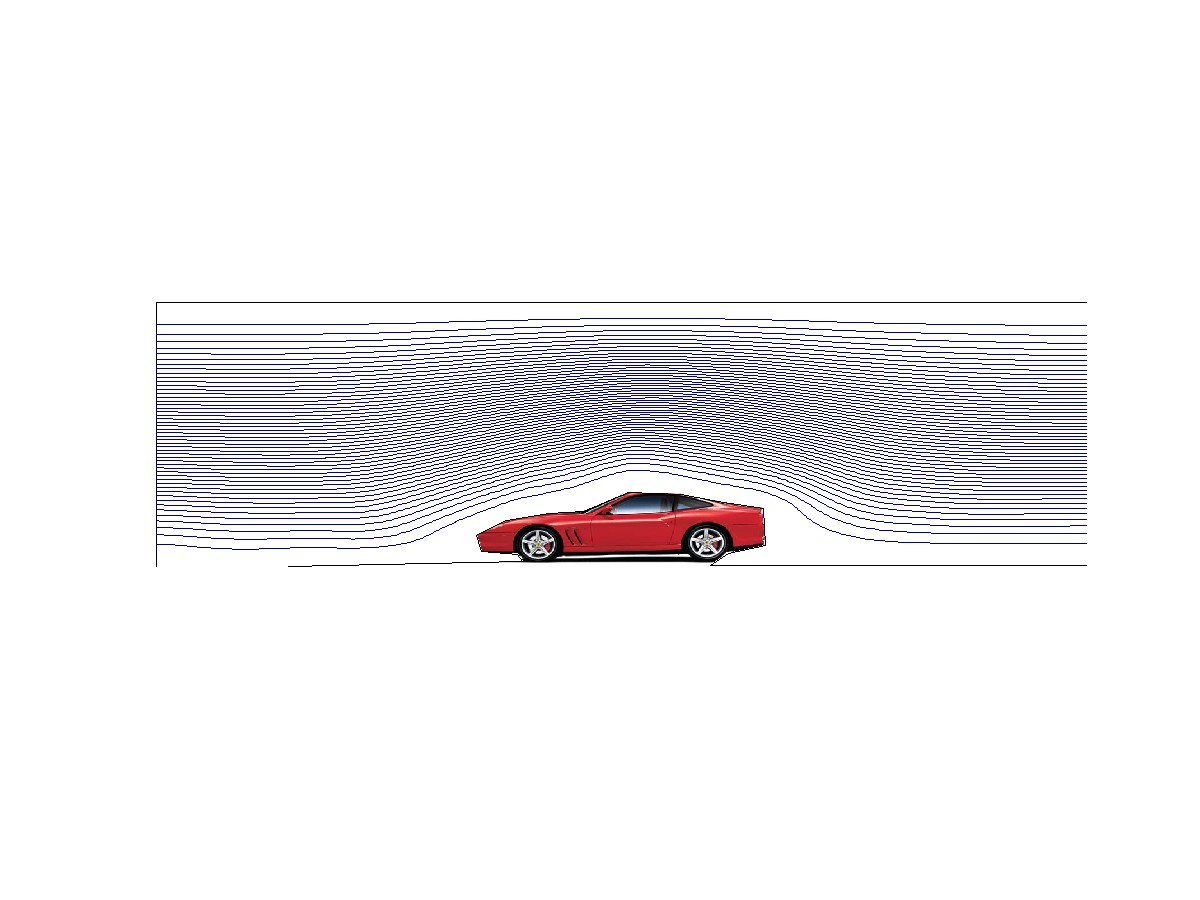
Flow Simulation based on Bivariate Splines
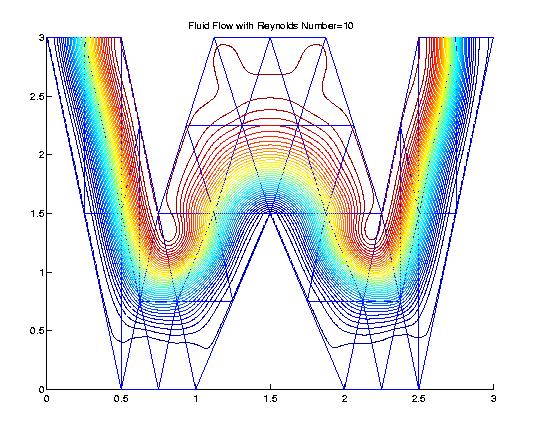
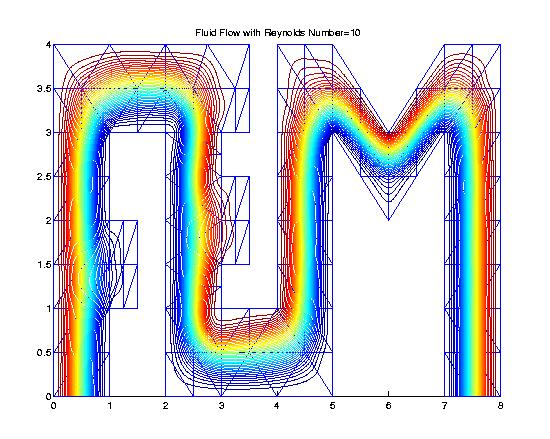
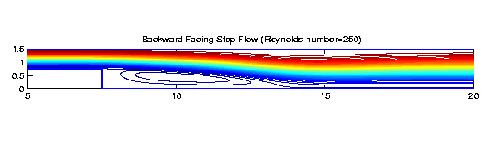
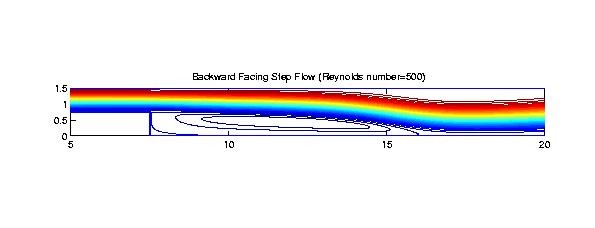
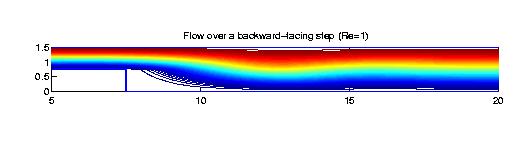
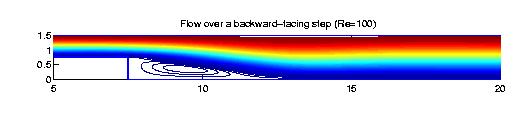
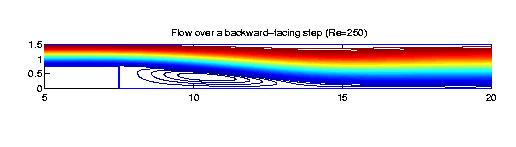
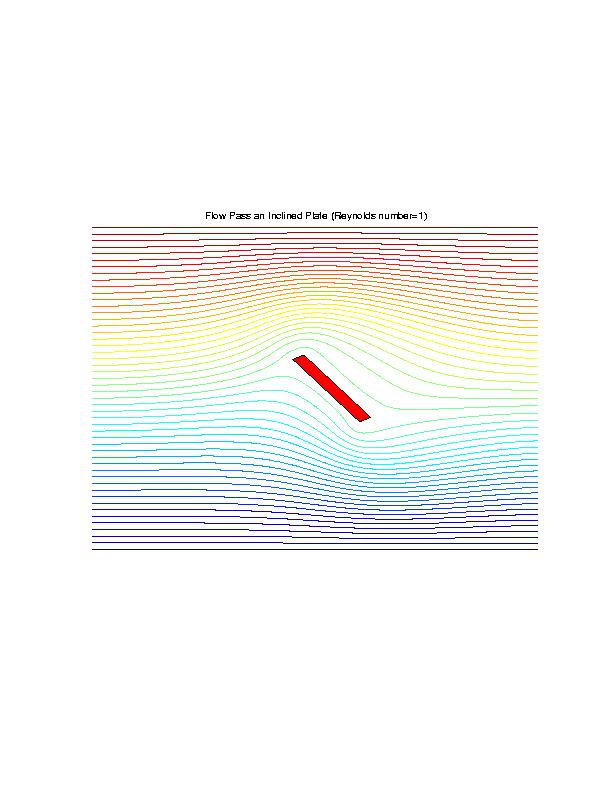
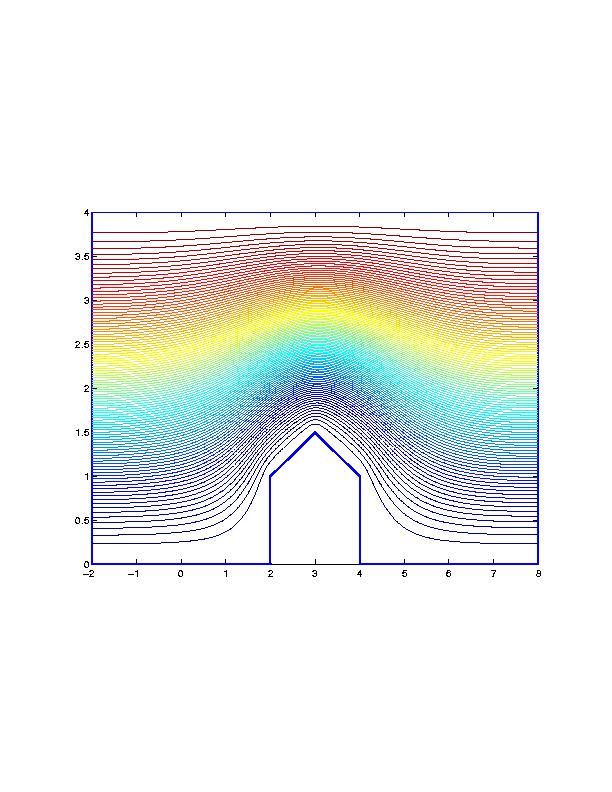
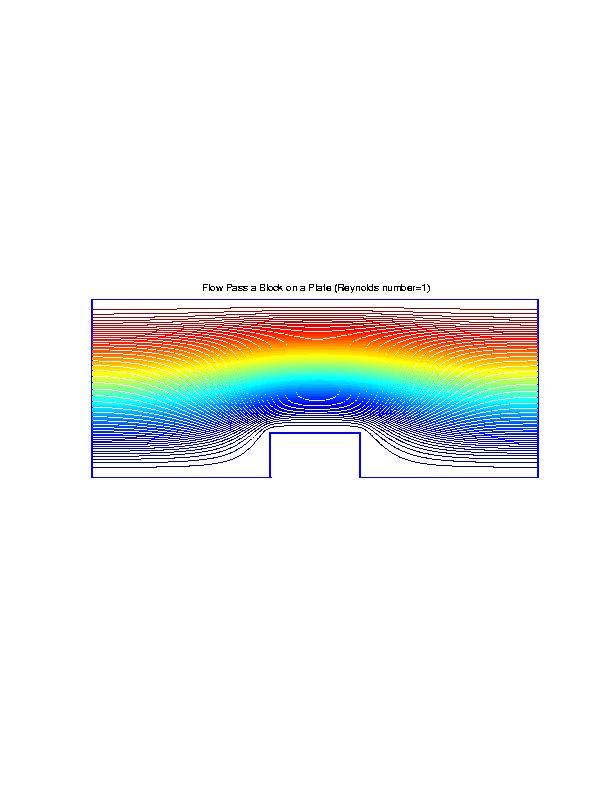
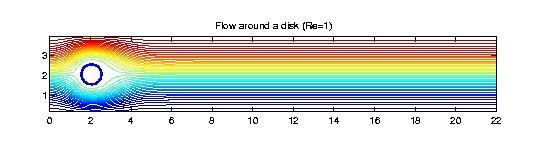
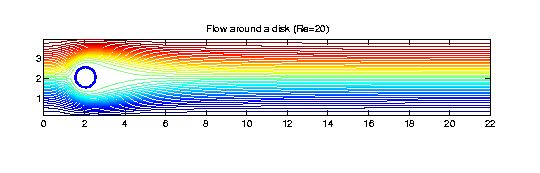
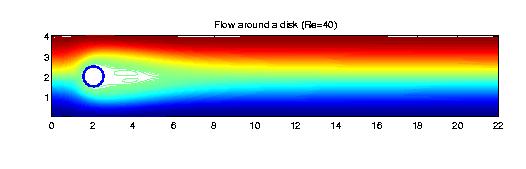
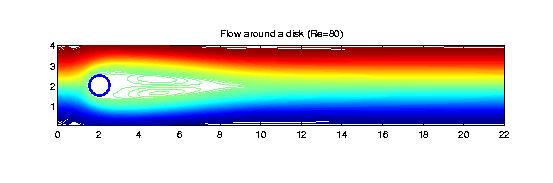
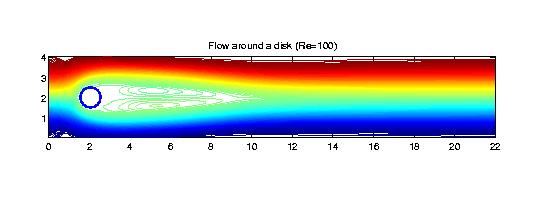
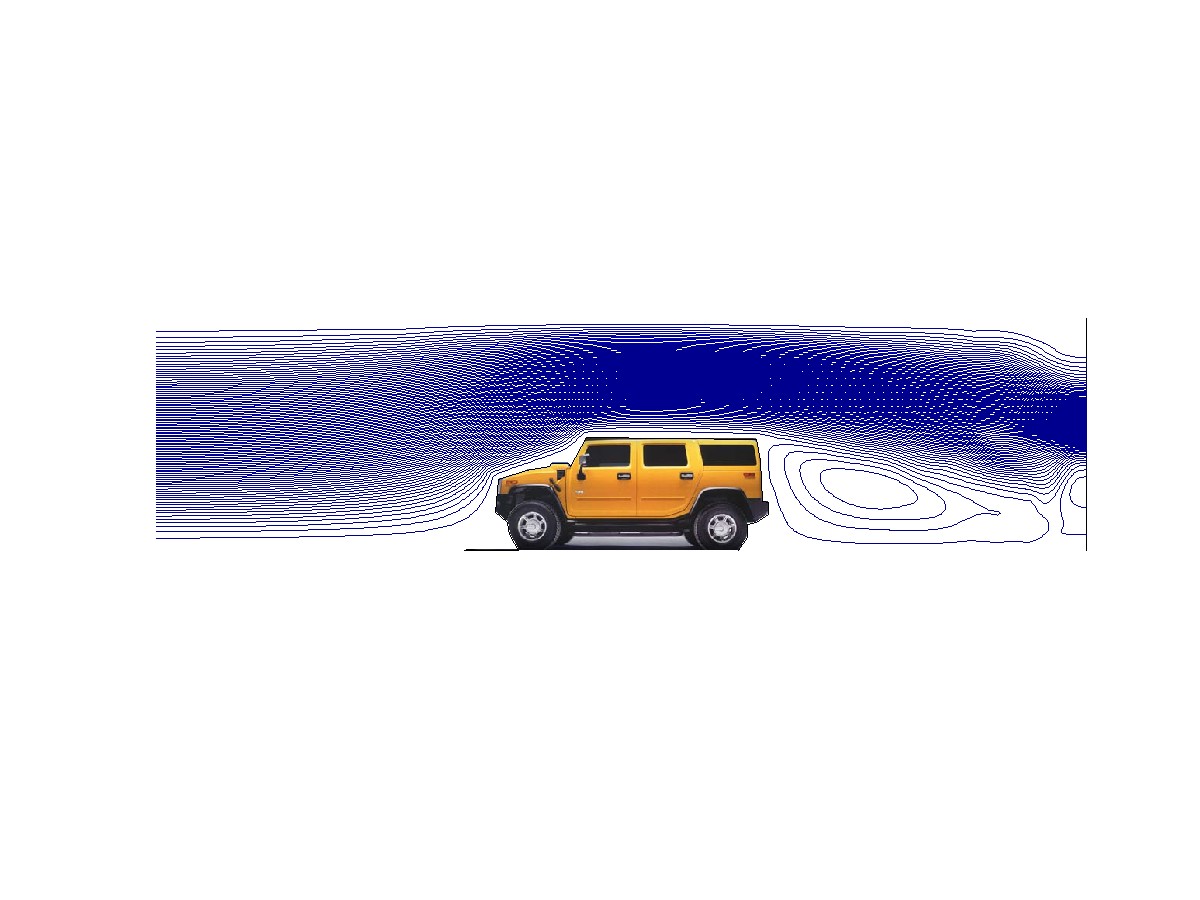
Fluid Flow Simulations We use bivariate splines to solve 2D steady-state Navier-Stokes equations. The main features of our methods are (1) the stream function formulation that is used so that the divergent free condition is satisfied exactly; (2) splines of higher degree and more smoothness are used so that the vorticity is a continuous function; (3) the solutions with higher Reynolds numbers can be computed easily (see examples below); (4) Navier-Stokes equations over arbitrary polygonal domains can be solved; (5) our methods are very efficient and effective. All the following numerical experiments were performed on a Sun workstation with 172 Mhz and a PC laptop with 400 Mhz. I did not use any supercomputers nor any parallel processes.
Cavity Driven Flow The following are standard cavity driven flows with various Reynolds numbers: 2000, 3000, 4000, 5000, 6000, 7000, 8000, 9000, 10000, 11000, 12000, 13000, 14000, 15000, 16000, 17000, 18000, 19000, 20000.
The vorticity of the cavity driven flows can be found here. 1, 100, 1000.